The determinants of propofol induction time in anesthesia
Article information
Abstract
Background
The required dose of anesthetics is generally smaller in patients with low cardiac output (CO). A high CO decreases the blood concentration of anesthetics during induction and maintenance of anesthesia. However, a high CO may also shorten the delivery time of anesthetics to the effect site, e.g. the brain. We assessed the time required for induction of anesthesia with propofol administered by target-controlled infusion (TCI), and investigated factors that modify the pharmacodynamics of propofol.
Methods
After measuring CO and blood volume (BV) by dye densitometry, propofol was infused using TCI to simulate a plasma concentration of 3 µg/ml. After infusion, the time taken to achieve bispectral index (BIS) values of 80 and 60 was determined. Age, sex, lean body mass (LBM), and cardiovascular parameters were analyzed as independent variables. The dependent variables were the time taken to achieve each BIS value and the plasma concentration of propofol (Cp) 10 min after the commencement of infusion.
Results
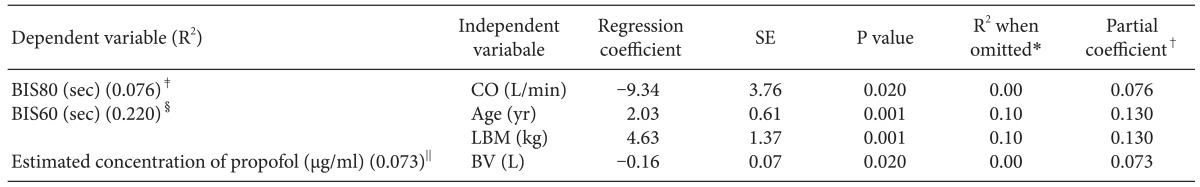
Multiple regression analysis revealed that a high CO significantly reduced the time taken to reach the first end point (P = 0.020, R2 = 0.076). Age and LBM significantly prolonged the time taken to reach the second end point (P = 0.001). Cp was negatively correlated with BV (P = 0.020, R2 = 0.073).
Conclusions
Cardiac output was a statistically significant factor for predicting the time required for induction of anesthesia in the initial phase, whereas, age and LBM were significant variables in the late phase. The pharmacodynamics of propofol was intricately altered by CO, age, and LBM.
Introduction
The hypnotic dose of propofol required for induction of anesthesia, including the type of infusion, has been extensively studied [1-5], and a series of factors that modify the dose required to achieve hypnosis have been reported [6-8]. In addition to patient-specific physiological variables affecting the pharmacokinetics of propofol, we previously demonstrated the effect of cardiac output (CO) on the hypnotic dose of propofol [1,2]. Cardiac output was a small but significant variable in predicting the hypnotic dose of propofol, time to hypnosis, and plasma concentration of propofol [8-10]. Other predictive variables were age and body weight [11,12].
The appearance of the hypnotic effect of propofol depends on its pharmacokinetics and pharmacodynamics [13,14], and CO is one of the parameters used to determined the pharmacological properties of propofol. In low CO patients, the blood concentration of propofol tends to be higher and the time to induction of hypnosis may be shorter [1,2]. However, there is a possibility that delivery of hypnotics into the brain may be more rapid in high CO patients because of its short circulatory transit time. Recently, a more practical and suitable drug administration technique, target-controlled infusion (TCI), has become popular in clinical settings. This new technique infuses a larger dose of propofol that is calculated to achieve a pharmacokinetic pseudo-steady state rapidly [5] and to attain hypnosis quickly. However, the effect of CO on the induction time when using propofol and TCI is not known. Thus, we hypothesized that large CO could distribute anesthetics to the target effector site more rapidly despite the slower increase of plasma concentration of propofol.
In the current investigation, we measured the time required to induce anesthesia with propofol administered using the TCI technique. We also investigated the effects of CO and other physiological factors on the pharmacodynamics of propofol.
Materials and Methods
The study was approved by the Division Ethical Committee of the Institutional Review Board. Written informed consent was obtained from 80 successively scheduled surgical patients who participated in the study. Parents of patients less than 20 years of age were asked to sign the consent form in addition to signing the consent form themselves. Patients younger than 12 years of age were not registered as participants. All patients required general anesthesia and oro-tracheal intubation, and were of American Society of Anesthesiologists physical status I or II. We excluded patients with severe cardiovascular disease, liver or renal dysfunction, lung disease, and patients treated with psychotropic agents.
Thirty min before entering the operating theatre, all patients were premedicated with hydroxyzine 1 mg/kg and atropine 10 µg/kg intramuscularly. Heart rate, electrocardiogram, noninvasive blood pressure, and pulse-oximetry were monitored. At the commencement of induction of anesthesia, 0.4 mg/kg of 0.25% indocyanine green (ICG) solution was administered into a peripheral vein over a few seconds, followed by purging with 20 ml of saline. The probe of the integrated pulse spectrophotometry monitoring system (DDG1001; Nihon Kohden Inc., Tokyo, Japan) was attached to the thumb of the patient [1,2]. The rate of plasma ICG disappearance, k [CICG(t) = CICG(0)·e-kt], was computed by linear regression from a semilogarithmic plot of ICG concentration versus time from approximately 2 min to 6 min after injection, where CICG(t) = ICG concentration at t minutes after injection and e = natural logarithm [15]. Measurement of CO and blood volume (BV) was usually completed within 6 min. Propofol was then intravenously infused to attain a steady plasma concentration of 3 µg/ml using the TCI technique based on the pharmacokinetic parameters introduced by Schnider et al. [5] (RUGLOOP®, version 3.14, DeSmet T. and Struys M. Department of Anesthesia, University Hospital Ghent). The calculating parameter included patient age, sex, body weight and height. Changes in EEGs were assessed using the bispectral index (BIS: A-1050, version 3.4, Aspect Medical Inc., Natick, MA, USA. The averaging window was 15 seconds). The time required to achieve BIS values of 80 (BIS80) and 60 (BIS60) were determined. Patients who showed BIS values less than 96 at the beginning of induction were excluded from the analysis. The displayed BIS values in the window were decreased and fluctuated, and therefore, BIS80 and BIS60 were defined as the times when the displaying number of BIS value first reached each of the end point values, 80 and 60. We hypothesized BIS80 as the point of a very light anesthetic and hypnotic state, and BIS60 as the point of an adequate anesthetic state, with reference to a previous investigation [12].
The venous plasma concentration of propofol was measured 10 min after the start of infusion [1] to prevent from invasive stimuli of venipuncture during induction and from measurement of BIS80 and BIS60. Three ml of whole blood was withdrawn from the patient's cubitus vein by brief puncturing. The sample was immediately centrifuged, and the separated plasma was extracted and frozen until analysis. The concentration was determined using high-performance liquid chromatography with fluorescence detection at 310 nm after excitation at 276 nm (RF550; Shimadsu, Kyoto, Japan). The areas under the chromatographic peaks were calculated with an integrator (PowerChrom; ADInstrument, Tokyo, Japan).
Age, sex, lean body mass (LBM), cardiovascular parameters (including CO, BV, and the rate of plasma ICG disappearance measured by dye-densitometry [k]), and the plasma concentration of propofol were analyzed as independent variables. The dependent variables were BIS80 and BIS60. The plasma concentration of propofol was analyzed as a dependent variable when age, sex, LBM, CO, BV and k were chosen as independent variables [1]. We applied McHenry's select algorithm to find the subset that provided the maximum value for R-squared (R2), and the correlation between each of the variables was calculated using a linear regression model. Subsequent step-up selection determined candidate variables for the model that increased the correlation the most, and we stopped adding independent variables when the remaining variables were not significant (P > 0.05). A value of P < 0.05 was considered statistically significant. Data are presented as the mean ± SD. Statistical analysis was performed with the software package NCSS 2000 (Number Cruncher Statistical Systems, Kaysville, UT).
Results
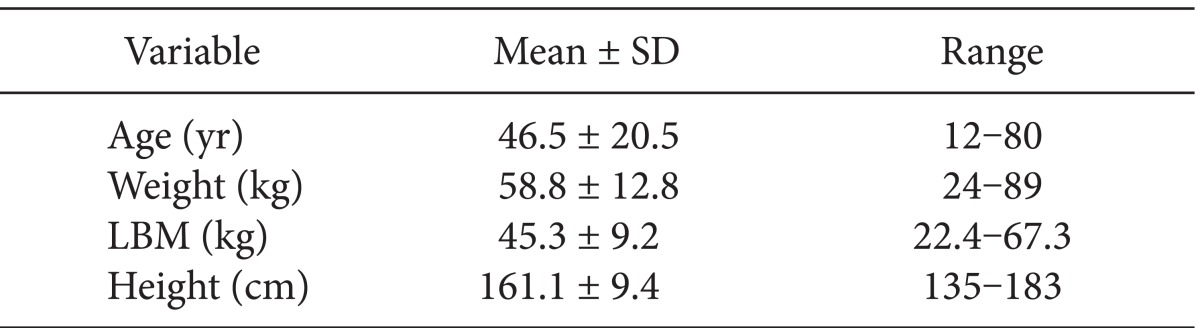
Complete data sets were obtained from all 80 patients. The demographic characteristics of the patients are shown in Table 1, and each measuring value is demonstrated in Table 2. Multiple regression analysis using a general linear model revealed that increased CO significantly shortened the time taken to reach BIS80 (R2 = 0.076, P < 0.020) (Table 3, Fig. 1). For BIS80, CO was determined as the only significant predictive variable. Increased age significantly prolonged the time taken to reach BIS60, as did increased LBM (R2 = 0.220, P < 0.001). The plasma concentration of propofol was not a predictive variable for the time required to achieve either end point, and it was lowered by increased BV (R2 = 0.073, P < 0.020) (Table 3, Fig. 1). Multicollinearity among independent variables was rejected in all models, because the variance inflation factor was < 10, and all the condition numbers for the eigenvalues of centered correlations were < 10. The power values of each accepted model were greater than 0.7.

The relationships between each independent and dependent variables. The regression lines were determined using a single linear regression for each relationship. (A) Cardiac output and the time to BIS80. (B) Age and time to BIS60. (C) Lean body mass and time to BIS60. (D) Blood volume and plasma concentration of propofol.
Discussion
Propofol was administered using the TCI technique during induction of anesthesia, and we determined the effects of CO and other physiological factors on the time required to achieve two end points, BIS80 and BIS60. Although there are slight fundamental and theoretical lines of evidence, we hypothesized that the primary end point may be considered to represent the attainment of hypnosis, and the secondary end point may be considered to represent the attainment of anesthesia, as signs of change in consciousness. The value of BIS60 in the result is approximately double that of BIS80 (303 vs. 163 sec as mean values, respectively), and the difference concerning predictive factors indicates a change in consequential factors depending on the phase of induction, whether early or late. Multiple regression analysis demonstrated that CO exerted a small but significant effect on the time taken to reach BIS80. Age and LBM markedly modulated the time required to reach BIS60. The plasma concentration of propofol was not a predictive variable for either end point, because the concentration may be influenced by BV at the end of induction rather than other factors [1].
At the commencement of induction of anesthesia, propofol administered through a peripheral vein circulates in the blood stream and is distributed to the whole body [4]. The drug may spread rapidly in patients with a high CO, and thus, infiltration to the target site might be achieved in a relatively short time. Previous investigations have shown that patients with a high CO required a larger dose of propofol and took a longer time to reach hypnosis [1,8]. However, in the current investigation, CO was determined to be a negative predictive variable for BIS80 in the regression model for the early phase. A high CO decreases the plasma concentration of administered propofol [1,2], although, increased CO may accelerate the delivery of the drug from the injection site to the target site [16,17]. In our previous study [1], propofol was infused slowly (15 mg/kg/hr) over 8 min. Thus, the influence of ke0 would be very small, and the pharmacokinetic effect could be acceptable for explaining the results. The profile of gradual pharmacokinetic increase in propofol concentration was different from that of rapid increase using the TCI technique. CO may demonstrate another pharmacodynamic effect of propofol, especially in the initial phase of induction.
In order to explore the effect of aging on the hypnotic activity of propofol, we selected the TCI protocol to simulate plasma concentration instead of effect site concentration. Age and LBM were selected as positive predictive variables for the time taken to achieve the second end point, BIS60. Elderly patients required a longer time to reach BIS60. Aging may prolong the time required for distribution to, or equilibration of, propofol at the effect site in the late phase of induction. Larsson and Wahlstrom [18] reported that there is an age-dependent development of acute tolerance to propofol [19]. However, using EEG analysis, age was reported to have no effect on the rate of BIS reduction related to increasing concentrations of propofol [12]. Furthermore, using a semilinear canonical correlation, Schnider et al. [11] reported that elderly patients were more sensitive to the hypnotic and EEG effects of propofol than were younger persons. Although the results for the effect of aging on the pharmacodynamics of propofol are inconsistent, it is accepted that the pharmacodynamics of the acute induction phase and pseudo-steady state are distinctive [20]. During induction, more physiological variables would modify the pharmacodynamics of propofol. We speculate that achieving equilibration at the effect site, or sensitivity to hypnotics, differs between older and younger patients [18,21]. In the late phase of induction, the pharmacodynamics of propofol may be affected by age. However, a possibility of statistical overestimation using multiple regression analysis would remain, because the factor of aging was distinguished after eliminating other interferences by mathematical calculations. There was a room for discussion regarding logicalness using Schnider's [5] algorithm against the simulating process concerning the effect of aging. Further investigation is required concerning this matter.
Schnider's pharmacokinetic parameters were based on LBM [5,11], and our previous investigation demonstrated the accuracy of pharmacokinetic predictions of these parameters [1]. The plasma concentration of propofol tends to increase in patients when continuous infusion of propofol is based on total body weight [22]. Furthermore, adjustment for LBM is recommended for appropriate administration of propofol [5,11,23]. Thus, the plasma concentration of propofol was expected be independent of LBM in the present study. However, LBM was a predictive variable for the time taken to reach BIS60, and patients with a higher LBM took longer to reach BIS60. We have no clear explanation for this result. One of the possible explanations is that the simulation based on conventional pharmacokinetic and pharmacodynamics parameters after achieving a steady state of anesthesia may be unsuitable during the progression phase of anesthesia. We speculated that not only the pharmacokinetic parameters, but also the pharmacodynamic parameterke0 would be different depending on the composition of body muscle and fat.
The plasma concentration of propofol was determined 10 min after the start of TCI. This is considered to be the least amount of time required to achieve a pseudo-steady state, and the concentration was correlated with BV only. The most optimized infusion regimen using the pharmacokinetic parameters of propofol based on LBM [5] minimized the variability of the plasma concentration. However, other physiological changes, e.g. dehydration caused by preoperative fasting, were able to modify the pharmacokinetics of the drug. BV may be correlated with LBM, but only BV showed a significant negative relationship with the plasma concentration of propofol. Although BV measurements by pulse dye densitometry were reported to have limited accuracy [24], BV was an important factor for the initial distribution volume of drugs, which was a consistent finding with that of our previous investigation [1]. The values of plasma concentration of propofol distributed between 1.38 to 5.56 µg/ml despite of the TCI set at 3 µg/ml. Precision and bias of TCI have been criticized from the beginning of the introduction of the method, and variation would become larger especially during induction of anesthesia [1,2,4,5,10]. However, we found a consistent and significant factor that affects the plasma concentration of propofol through multivariate analysis.
There are several potential limitations in the design and results of this investigation. The time taken to achieve either end point was defined as the earliest time at which each BIS value was first attained, and not as the time at which the BIS value became constant [12,25]. However, BIS values can undergo a linear-like decrease during the initial induction period [12]. Thus, the method used to assess the time to attain each BIS end point value may be acceptable. Statistical significance (P < 0.05) and a sufficient power value (Power > 0.7) for certain factors were obtained in the linear model multiple regression analysis used to confirm our results. The R2 value was rather small, except in the case of BIS60 in spite of a proper set of significant parameters and multivariable feedback control analysis used to calculate the biggest R2 value. The usefulness of linear regression for the type of data that we explored would be limited [26,27]. It was only BV and not LBM that significantly affected the time taken to reach BIS60. However, it is possible that both variables may be correlated since our results leave a room for discussion concerning statistical completeness. The Cardiac Index (CI) rather than CO may be a preferable factor in the analysis; however, we had already explored factors including patient weight and height. CI is a product of weight, height and CO, and we selected CO as an appropriate and independent variable to elude the statistical problem of multicollinearity.
The last limitation of the current investigation may be that we used the early developed apparatus for monitoring BIS and dye-densitometry. Although each version was not the latest one, the fundamental measurements and the analyzing process are almost identical with the current version, and the accuracy of the results was not influenced.
In summary, CO was a small but statistically significant factor in predicting the time required to reach the initial end point of hypnosis during induction of anesthesia with propofol. The results indicated that patients with a low cardiac output, older age, and with a larger LBM showed a longer time to achieve a hypnotic effect with propofol using TCI.
Acknowledgments
We express sincere thanks to Harue Kuge, Shizuko Kaneda, Kaai Mishima, Mikiko Ohbayashi and Sachiyo Tamura for their assistance in this investigation in the operating room.